Line spectra of "electrified" gases and Bohr's quantum theory of the atom

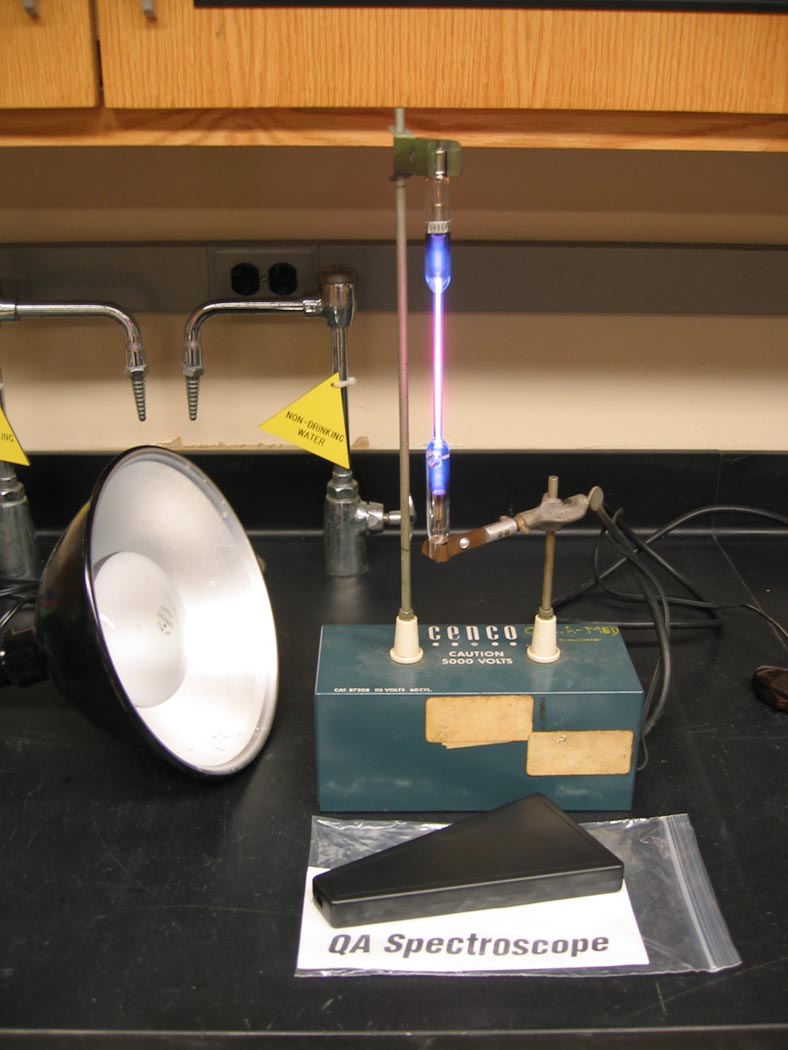Electrified tubes of gas are observed using an spectroscope; the spectra are found to be composed of spectral "lines" rather than continuous bands of color.

Ingredients: emission tubes, spectroscope

Procedure: A complete recipe follows.

1. Observe emissions of hydrogen gas through spectroscope.

2. Observe and compare deuterium gas emissions.

3. Observe emissions from nitrogen gas.

4. Observe and compare other multi-electron atom emissions, including neon and mercury.

Understanding: In observing the emissions of a hot black body, we noted that the spectra consisted of continuous bands of color covering the full visible spectrum. In observing the emissions of hydrogen gas, we are struck by the fact that they consist of discrete spectral lines. Such atomic line spectra were carefully analyzed in the late nineteenth century, leading to a number of empirical mathematical relations that successfully defined the observed frequencies of the spectral emissions of the hydrogen atom.

Subsets of spectral lines for the emissions of the hydrogen atom were isolated in different parts of the electromagnetic spectrum. In the UV region, a series of spectral lines forming the Lyman series were found to be related by the simple mathematical formula

ν = 3.29 x 1015 Hz (1 - 1/n2)

In this formula, n is a counting number that can take on the values n = 2, 3, 4, 5... where each value of n represents a particular spectral line. Values of n are restricted to be greater than or equal to 2 so that the frequencies are all positive.

In the visible region of the electromagnetic spectrum, a series of emission lines form the Balmer series

ν = 3.29 x 1015 Hz (1/4 - 1/n2)

where n = 3, 4, 5... restricted to values greater than or equal to 3. And in the IR region of the electromagnetic spectrum is found the Paschen series

ν = 3.29 x 1015 Hz (1/9 - 1/n2)

where n = 4, 5... defines the observed spectral emissions.

And the series go on! There is also the Brackett series

ν = 3.29 x 1015 Hz (1/16 - 1/n2)

where n= 5, 6 ... and the Pfund series

ν = 3.29 x 1015 Hz (1/25 - 1/n2)

where n= 6, 7... and the list could continue on to infinity. Clearly, there is a series of series of spectral lines. The question is, how can we understand these series of spectral emissions in terms of the dynamics of the electron and proton that form the hydrogen atom?

Niels Bohr builds a "planetary model" of the one-electron atom

Niels Bohr considered these spectral series and realized that he could explain all of the results using a simple planetary model of the hydrogen atom, and a few radical assumptions.

Let's consider a one electron atom or ion that has Z protons in the nucleus and one electron. Bohr's simple planetary model of the atom assumes that electrons follow circular orbits centered on the nucleus. The total energy of the atom is the sum of the potential energy, for the electrostatic attraction between the proton and electron, and the kinetic energy, for the motion of the electron

E = T + U = mv2/2 - Ze2/(4πε0r)

where Ze is the positive charge on the nucleus and -e is the negative charge on the electron.

There are two forces acting on the orbiting electron. The inward pointing centripetal force is due to the electrostatic attraction of the electron to the proton nucleus

Fc'petal = -dU(r)/dr = - Ze2/(4πε0r2)

The outward pointing centrifugal force is due to the ever turning and ever accelerating electron

Fc'fugal = mv2/r

For the electron to form a stable orbit about the nucleus, the inward pointing centripetal force must exactly balance the outward pointing centrifugal force. If we set the two forces to be equal in magnitude so that -Fc'petal=Fc'fugal we find the simple equality

Ze2/(4πε0r2) = mv2/r

This relation tells us that for a particular value of the radius, r, and centripetal force, there must be a particular velocity, v, leading to a centrifugal force that exactly balances the centripetal force, leading to a stable orbit of the electron. Fixing the radius of the orbit determines the velocity; fixing the velocity of the electron fixes the radius of the orbit.

This relation leads to another important result. If we multiply both sides by one-half and by r, we find

Ze2/(8πε0r) = mv2/2

The kinetic energy of the electron is equal to one-half the magnitude of the potential energy of the atom, T=-U/2. We then find that

E = T + U = U/2 = -Ze2/(8πε0r)

This result for the total energy of the atom is independent of the velocity of the electron. Why? Because once we determine the radius of the orbit of the electron, the velocity must take on a particular value so that the centrifugal force exactly balances the electrostatic force, leading to a stable orbit.

Niels Bohr makes some crazy conjectures to fit the experimental data

The classical theories stated that an accelerating charge would radiate energy. That would mean that by the classical laws of physics, Bohr's orbiting electron would forever radiate energy and spiral into the nucleus. Bohr said to ignore that idea; in his model, the electrons would execute stable, circular orbits forever unless they emitted or absorbed light energy.

To fit the discrete frequencies observed in the line spectra of hydrogen, Bohr knew that he needed to restrict the hydrogen atom to discrete energies of transition between initial and final states. He enforced that idea by assuming that the electrons could assume only certain values of the angular momentum

mvr = nh/2π

where h is Planck's constant and n=1,2,3, ... is a counting number that defines the discrete values of the angular momentum, mvr. Why was that justified? At the time there was no good physical reason. But Bohr knew that if he did enforce that constraint on the angular momentum, his theory would lead to agreement with experiment. Let's see how that works out.

In balancing the centrifugal and centripetal forces, we found that for every value of the radius there was a particular value of the velocity that produced a stable orbit. If we substitute that result

Ze2/(8πε0r) = mv2/2

in Bohr's formula for the angular momentum, and solve for r, we find that the atoms may assume only specific well-defined radii

rn = a0 (n2/Z)

where Z is the atomic number, the number of protons in the nucleus. Evaluating

a0 = ε0 h2/ π m e2 = 5.28 x 10-10 m

known as the Bohr radius.

As the atomic number increases, the charge on the nucleus increases and the orbits become smaller. That makes sense! And note that the allowed orbits take on only certain discrete radii, defined by the value of the quantum number, n.

We found that the energy of our atom was determined by the radius of the orbit of the electron. If we substitute the allowed radii into our result for the total energy

E = -Ze2/(8πε0r)

we find that for each allowed orbit of well-defined radius there is a well-defined energy

En = - Ry (Z2/n2)

where

Ry = m e4/(8 ε02 h2) = 2.18 x 10-18 J

using m=9.1 x 10-31 kg, e=1.60 x 10-19C, and ε0 = 8.85 x 10-12 C2/(J m). Ry is known as a Rydberg of energy.

In the n = ∞ state, the potential energy of the atom is zero and the electron is free to leave the nucleus. The energy associated with the transition in the one electron atom from the ground state, n = 1, to the state n = ∞ is the ionization energy of the atom, IE = RyZ2.

Emission and absorption spectra viewed through Bohr's model of the atom

Bohr found that his atom would have only certain discrete allowed orbits, each having well-defined radius and energy, determined by the value of the quantum number, n. If the atom emitted light, it must be that it made a transition from a higher energy orbit to a lower energy orbit. The energy of the emitted photon of light would be determined by the conservation of energy

Eatom before = Ephoton + Eatom after

It follows that the frequency of the emitted light is

ν = (Efinal - Einitial)/h = 3.29 x 1015 Hz (1/nf2 - 1/ni2)

Since the energy of the atom decreases when light is emitted, ni > nf. It turns out that the formula derived by Bohr fit the spectral series perfectly! Take the Lyman series

ν = 3.29 x 1015 Hz (1 - 1/n2)

Bohr found that he could set nf=1 and let ni be any value greater than 1. That means that the Lyman series was due to atoms in an excited state with quantum number ni=2, 3, 4... relaxing in energy to the ground state where nf=1.

To derive the Balmer series, he set nf=2. The emission lines of the Balmer series correspond to the hydrogen atom initially in an excited state with ni > 2 relaxing to a final state with nf=2. To derive the Paschen series, he set nf=3. And so on.

For the absorption of light, we can apply the conservation of energy to find

Eatom before + Ephoton = Eatom after

It follows that the frequency of the absorbed light is

ν = (Efinal - Einitial)/h = 3.29 x 1015 Hz (1/ni2 - 1/nf2)

Since the energy of the atom increases when light is emitted, ni < nf.

Exploring the Lyman, Balmer, and Paschen spectral series

Question: Using the result for the allowed transitions in the hydrogen atom, compute the full range of wavelengths of light for the absorption lines in the Lyman series. Note that the largest energy of transition is between the ground state, ni = 1, and the ionized state, where nf = ∞.

Repeat your calculation for the Balmer series and the Paschen series. In each case, identify the region of the electromagnetic spectrum in which the series is centered.

You can check your answers here.